
| ||||||||
Research Design and Analysis for Case Y
This page was written by Alastair Reynolds
This piece of research is set in the mathematics department of School Y, a selective boys’ grammar school. The department recognises that ICT can be beneficial in encouraging greater understanding of certain mathematical topics, and consequently ICT has become an integral part of the scheme of work in year eight. For two thirds of the year pupils are allocated one maths lesson a week using ICT facilities. Other years also have experience of learning using ICT with various applications.
This research is concerned with one of the year eight activities involving circles and LOGO. The aim of the research is to consider the effectiveness of the teaching and learning in this activity. A full description of the activity is given later in this page.
At the time of writing, all of year eight had already completed the task, so a group nearing the end of year seven were selected to try the task. They had covered the necessary background work earlier in the year. The group were of mixed ability, and had used the ICT facilities at various times during the year.
Access issues
Permission to conduct the research with the class was sought from the head of department and the teacher of the class. The nature and purpose of the research were explained to the pupils, and they were given the opportunity not to participate if they so wished. Permission to access departmental and school policy documentation was also established.
Ethical issues
All the parties involved in the research were made aware that the research was taking place. Participants were assured that anonymity would be maintained. In the case of pupils filling out worksheets before and after the activity, it was explained that the names on the sheets would only be used to match up the sheets, and that no record would be kept of the responses made by any individual pupil. Permission was also obtained for inclusion in this report of the research results, questionnaire responses and relevant departmental documentation.
Theoretical background
The activity is based around the relationship between the circumference, C, and the diameter, D, of a circle. Pupils are taught the formula C = pi × D, and learn to use this formula to find a circumference given a certain diameter (pi is approximately equal to 3.14). The other important circle relationship is between the area, A, and the radius, R, which is represented by the formula A = pi × R².

A problem arises because pupils remember the two formulae pi × D and pi × R² but often confuse their purposes, using the first to calculate area and vice versa. This is in part due to a lack of understanding of the nature of the relationship - that the circumference of a circle is approximately three times its diameter. The main purpose of the activity described below is to reinforce the understanding of that relationship.
Using LOGO to reinforce the relationship between circumference and diameter
The investigation is framed in terms of a task to discover the value of the constant pi. Early mathematicians achieved this by constructing circles, measuring their circumference and diameter as accurately as possible, and dividing the circumference by the diameter to get an estimate of pi. The pupils will follow a similar process, but rather than using pencil and paper, they will use the LOGO system as their workspace.
LOGO is actually a programming language but its facilities for geometric construction, often called “turtle graphics” features, are most used. Because the package is restricted to straight lines, there is no potential for construction of circles. Regular polygons can be used to approximate circles, and appear more like circles as the number of sides is increased. For example, a polygon with 5 sides of length 100 and a polygon with 50 sides of length 10 will both have perimeter 500, but the latter will look like a circle.
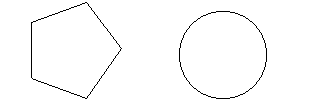
The pupils will be asked to construct several regular polygons, calculating the perimeter and measuring the distance across the middle in each case. They will then use these figures to calculate estimates of pi. The distance across the middle is found by moving the LOGO “pointer” across the centre of the polygon until the other side is reached - a process of trial and improvement. To aid their initial estimation, a routine to draw a scale on the screen is provided.
An HTML copy of the teacher's guide to the activity may be found in Appendix E.
An HTML copy of the activity sheet used by the pupils may be found in Appendix F.
The study is intended to discover whether the activity described above is effective in achieving its primary objective; to reinforce the pupils’ understanding of the circumference-diameter relationship. To do this a variety of data collection techniques were used.
Pre-activity and post-activity pupil questionnaires
To gain an idea of the change in pupils’ knowledge as a result of the activity, each pupil was asked to fill out three questionnaires. These were completed before the activity, directly after the activity, and approximately two weeks later. They should allow some analysis of the short and longer-term effects of the activity.
The three questionnaires had a similar structure, asking five questions. The first two questions were factual, asking pupils to name two parts of the circle. The next two questions were intended to test procedural understanding, asking pupils to estimate a circumference given a diameter and vice versa. The final question was a conceptual one, asking pupils to describe (in words or symbols) the relationship between the circumference and diameter.
An HTML copy of one of the pupil questionnaires may be found in Appendix G.
Post-activity staff questionnaires
Those teachers who had conducted a similar activity with year eight classes were asked to complete a questionnaire about the task. The results of these will be used to see whether the intended aims and effects of the activity were the same as those perceived by the teachers. The questions included were:
Direct observation of pupils
During the course of the activity, the teacher interacted with the pupils, and recorded significant events or noteworthy pieces of dialogue. The aim of this was to gain insight into the learning processes and to provide a more descriptive aspect to the research results.
Data Analysis
The analysis will be primarily qualitive; the results of the various questionnaires and observations will be collated and examined. The aim will be to determine whether the activity was effective in achieving its objectives, and reference will be made to the work on effectiveness discussed in the literature review. The possible reasons why the activity was or was not effective will also be considered at this stage. Although numerical analysis could be performed on the results of the pupil questionnaires, it would be unwise to draw conclusions from a sample of this size.
Direct observation of pupils
The session went well. Although the time available was slightly too short, the pupils got involved with the task quickly and most were clear on what they were supposed to do. Pupils worked in pairs and this was useful in rectifying initial misconceptions about the task. Because pupils had already experienced the use of IT in learning Maths, they were familiar with the machines and software; the task was seen as part of the normal flow of teaching rather than a “novelty” activity.
Most of the conversations between pupils were planning what values to try next, choosing number and length of sides. The teacher approached pairs of pupils, asking questions designed to get the pupils thinking about the relationships (e.g. How do you know that diameter value is about right?). Some pairs clearly developed ways of estimating the ‘diameter’. When questioned they were able to explain that the distance across the middle was always about a third of the perimeter of the polygon, and they had simply divided the perimeter by three to get their first estimate. Other pairs carried out the task without this realisation, using the scale to provide an initial estimate of the diameter throughout.
Pupil questionnaires
The questions on the pupil questionnaires were deliberately designed to test different types of understanding. The first two questions were about facts relating to circles. The third and fourth were about procedures for working with the circumference and diameter of a circle. The final question was about the conceptual relationship between the diameter and the circumference.
In the pre-activity questionnaire, there were very few incorrect answers to the first two questions. The topic was taught earlier in the year and clearly this information had been retained by most pupils. There were no incorrect answers to these questions in the questionnaires taken immediately after the activity and two weeks later.
Before the activity, only six pupils (of twenty-three) answered the third and fourth questions with any degree of success. Immediately after the activity, almost every pupil answered these correctly (although they tended to use the value 3 rather than pi). The results in the questionnaire two weeks later were similar; only two pupils were unable to answer correctly.
The fifth question posed the most problems. Only four pupils gave correct answers before the activity. Twelve pupils gave correct answers to the questionnaire immediately after the activity, and ten answered correctly in the questionnaire two weeks later.
The numerical results of the pupil questionnaires can be found in Appendix H.
Staff questionnaires
The staff questionnaire was intended to see whether other teachers’ objectives for this task were the same as the intended objectives, and whether they percieved effective learning.
All four staff included pupils estimating pi as an aim of the task. Three staff included the reinforcement of the diameter-circumference relationship, which was the stated aim. All four staff felt that the aims they stated had been achieved, but two commented that pupils might not appreciate what they had learnt. This was felt to be because the teacher’s learning objectives were not made explicit at any point during the activity.
There was a general feeling that the amount of knowledge which would be retained by pupils would vary greatly; that some pupils would not retain much of the knowledge gained. It was interesting to note, however, that almost all of those pupils who gained new skills or understanding as a result of the activity could still recall that new understanding two weeks later.
All the staff recognised that the task was enjoyable to most pupils.
A summary of the responses to the staff questionnaire can be found in Appendix J.
Research results
The teacher interaction with pupils during the task proved to be important in developing pupils understanding of the circle relationship. Questioning of the kind described in Scrimshaw (1993) encouraged pupils to analyse more carefully their own decision-making and checking procedures.
The LOGO components of the task were only tools in each experiment, but the ease with which experiments could be repeated and varied led to a more fluid investigative environment. Pupils did not get bored because the repetitive tasks were being carried out by the computer.
The results of the pupil questionnaires were encouraging. Most pupils gained an understanding of how circumference can be estimated from diameter and vice versa, and were able to recall this understanding two weeks later. There was less success in the description of the theoretical relationship between circumference and diameter. Pupils’ success in the questions examining procedural understanding seems to indicate that it was an inability to communicate the relationship which led to lower performance in the fifth question.
It was interesting to note that all four staff included estimation of pi as an aim for the task. It is certainly the stated aim as far as pupils are concerned, and it gives the task a higher level of authenticity since Greek Mathematicians attempted to find estimates of pi in this way. Davis et al (1997) would argue that this was authenticity to the subject, rather than to a real-life context.
There was a general feeling from the staff that the amount of work which would be retained would depend on the individual pupil. This was interesting since the majority of those pupils who gained new skills or understanding as a result of the activity could still recall that new understanding two weeks later.
Style of learning
It is hard to identify the learning model which is most applicable to this activity. It appears that different pupils are learning in different ways.
Some pupils have formed a conceptual understanding of the relationship between the circumference and diameter. These pupils were able to describe the relationship using words or symbols. This has not been a result of the activity alone; teacher input was crucial to the process. This is an example of scaffolding - the task and teacher intervention assist the pupil in building their cognitive schema.
Aspects of the task appear to encourage behaviourist learning; the pupil repeats the experiment and always finds that the diameter is approximately one third of the circumference. This is like a stimulus-response pair in some respects. The activity is not as structured as most behaviourist tasks, however, and there is no clear evidence that pupils are learning the response without any intermediate cognitive processing.
Perhaps the most appropriate learning model in this case is apprenticeship. All the pupils developed some skill in estimating diameters from circumferences as a result of the activity, and that skill was retained at a later date. The argument against this is that the skills they developed were not demonstrated to them, except by other pupils who had already acquired the skills.
Since the intended outcomes of the task included the development of both procedural and conceptual knowledge, it should not be surprising that the main types of learning should be apprenticeship and constructionist. The relative success of pupils in those questions which tested skills indicates that most pupils experienced apprenticeship learning, and a smaller proportion experienced constructionist learning.
The activity was effective in some respects. All the pupils gained some skills in using the circumference-diameter relationship to answer questions about circles. Half the pupils were able to clearly describe the relationship after the task. Most pupils who gained new knowledge retained that knowledge when questioned two weeks after the activity.
There are a variety of factors which could have led to the activity being effective :
Through this assignment I have gained a much clearer understanding of the importance of the literature review. It has influenced the design of the research and has provided a reference point in educational theory with which my results could be compared. Much of the value of this assignment is in the knowledge and understanding I have gained through researching and reading the various texts pertaining to this subject.
I have gained a greater appreciation of the value of designing a research method after reviewing the literature and before conducting the research. Considering the methods of data collection and analysis in advance meant that more meaningful conclusions could be drawn.
At times I was surprised to discover that the activity I was considering was actually achieving its aims. The use of evidence from a variety of sources was important to my realisation of this fact, and I have become more aware of the need for evidence rather than ‘gut feelings’ when doing educational research. I think that a balance between quantitative and qualitative approaches allows the reader of any research to make more informed judgements about the validity of any conclusions.
You may now wish to go to the Case Study X page if you have not already read it.
Otherwise you can move on to the
combined conclusions or return to the
contents.